This week, we are going to touch on a more complicated concept than usual. I’d like to talk about music boxes and, as an extension, lay down the groundwork for acoustics in general. For any Music and Technology majors or musicians reading, I apologize if I botch anything here. But why talk about music in the first place? Music has always had a very important impact on my life. I often use it as an escape. I know that when I don’t know what to do—when I’ve exhausted every option and given all I can — I can still listen to music. For me, music is feeling. Sometimes, I feel it in my head or chest, sometimes in the tips of my fingers or my ears, and sometimes in my heart. There are few feelings better than resonating with a particular song or sound — feeling seen by another human being or being in a way that transcends familiarity.
But just what is resonance, and what does it have to do with music? Let’s start with the basics. Sound, as we know it, is the vibration of air molecules that vibrates our eardrums. Our brain detects those vibrations and converts them into electrical signals we understand as sound. Vibrations are oscillations, or when something or a group of things move back and forth over a certain period of time. Vibrations can be described by their frequency, which is the unit Hertz, which is the number of cycles per second. In other words, vibrations with high frequencies are moving back and forth very quickly, and those with low frequencies are moving back and forth very slowly. Every object has a natural frequency. This is the frequency at which that object will tend to vibrate when struck by something, or in the case of a guitar string plucked. Aside from its natural frequency, objects also have other special frequencies called resonant frequencies. These resonant frequencies are special because objects take on particular patterns of vibration when they vibrate at them, called mode shapes. These mode shapes contain a lot of energy, and the size of the vibrations is much larger than at frequencies other than these natural frequencies.
With these concepts outlined, let’s return to music boxes. The natural frequency of an object is dependent on things like its stiffness, mass, and geometry. A music box has a row of metal bars with one end attached to a plate and the other end resting on a cylinder with bumps. As the cylinder spins, the bumps pluck each of the bars in a specific order. The different sounds are dictated by the natural frequencies that these bars vibrate at, which differ between them due to their differing lengths. When the bars vibrate, they vibrate the air around them, which eventually vibrates our eardrums, allowing us to hear them. Guitar strings function in a similar way, but their natural frequencies differ depending on their thickness and how tightly they are attached to the guitar. In an acoustic guitar, the strings themselves don’t move much air or produce a lot of sound. The guitar body is shaped in such a way that the vibrations of the strings vibrate the top of the guitar, and the air inside the guitar resonates and amplifies the sound. Different guitar shapes consequently emphasize different notes and sound distinct due to the resonant frequencies of their bodies.
I think that’s what makes music so beautiful; there is something fundamental about vibrations. On a small enough scale, everything in existence is oscillating in some way or another, like waves of light. In that sense, the universe sings to us all, and we are each an instrument in this cosmic choir. Find those with whom you resonate, and you will be capable of more than you ever thought possible.
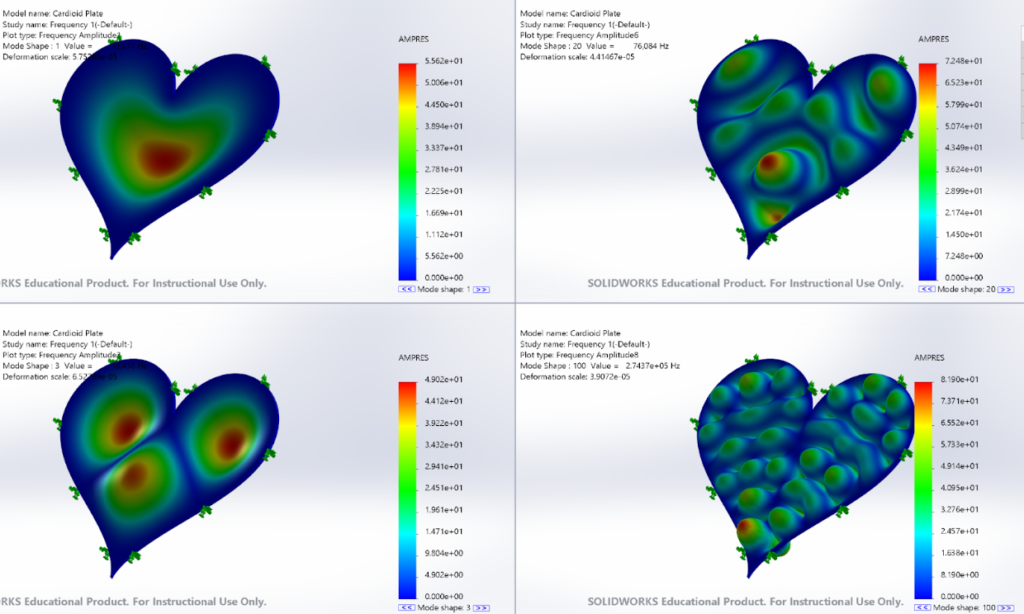
Not sure if it can be included in the article, but a couple of mode shapes for a thin, heart-shaped plate fixed at its boundary. Curves are driven by the parametric equations:
x = 16 cos3(t), y =13 cos(t)-5 cos(2 t)-2 cos(3 t) -cos(4 t), 0≤t≤2π (rad)
If anybody who is actually reading this is interested, I can send more mode shapes upon request. Have a good day :^]