(shot by James L. Amos)
Mathematicians love tiling, the process of covering a surface using some set of geometric shapes with no gaps and overlaps. Tiling or tessellations can be seen in everything from art, to architecture, to even nature. The repeating hexagon in a bees honeycomb, an ancient Roman mosaic, and even the tiles in your bathroom, are all examples of a repeated shape that completely covers the plane.
A great deal of research in math has been on periodic and aperiodic tilings: patterns of shapes that repeat and do not repeat, respectively. A famous example is Penrose tiling, a class of shapes that do not repeat, but can have rotational and reflection symmetry. Just last year, researchers developed a shape called an ‘einstein’ or an aperiodic monotile, a shape that tiles the entire plane, but never repeats. This research into tiling has continued, with a new discovery very recently.
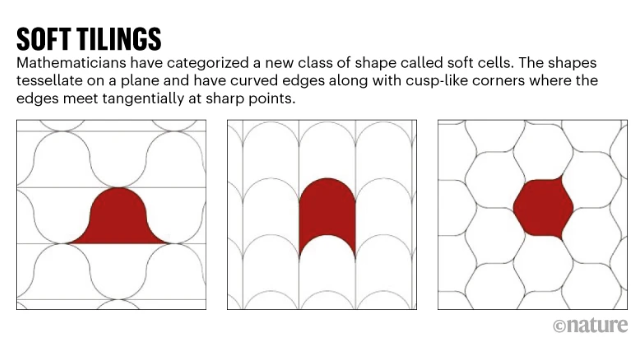
Researchers at the University of Oxford and Budapest University of Technology and Economics have recently described an entirely new class of shapes: soft cells. These shapes have a soft number of edges that are smooth, with two cusp corners that add sides to the shape. The corners meet at kind of a ‘teardrop’ shape, and are rounded on the sides, with the sum of the interior angles being zero and can fit snugly together in a tiling pattern.
In order to create this different class of shapes, researchers created an algorithm that took conventional geometric tiles, like polygons, and converted them into soft shells. This also led to them developing a measure of how ‘soft’ her shapes are, or how rounded the edges are. These shapes can even be converted into 3D tilings, which is done by taking a 3D shape and converting it into a soft shell with two cusps.
Since the discovery, these shapes can be seen everywhere. The nautilus shell, already famous in math for using the Fibonacci sequence, has also been found to have a 3D soft shell shape in part of its segment. Soft shells can also be seen in the way onion layers form, or in the way rivers seem to braid around land. It can be found in architecture. For example, the Heydar Aliyev Center in Baku, Azerbaijan, uses cusps to minimize corners. Soft shells also appear in biological tissue, which adds new insights into biology and geometry research.
Further research will look more into the ‘softness’ factor of the shape, with mathematicians predicting that for a given tiling, there is a unique shape with the greatest possible softness. Researchers will also look for materials that utilize this tiling, with the hope of illuminating how these shapes can affect measures such as bending or tension. This new insight into nature provides researchers with an interesting connection between several different fields, and as Alain Goriely, a professor at the University of Oxford who worked on the project, put it: “Nature not only abhors a vacuum but she also seems to abhor sharp corners.”